This polytope lives in the four dimensionnal space R⁴. Two of its names are the Runcinated 120-cell and the Runcinated 600-cell. It is Archimedean in the sense that its organization at every vertex is the same, and all its chambers are Archimedean polyhedra too: at each vertex, one finds a regular dodecahedron, three prisms with regular pentagonal base and square sides, three prisms with equilateral triangular base and square sides, and a regular tetrahedron.

Here are some statistics about the object:

It has 2400 vertices (a.k.a. 0D-cells), they all lie a the same distance from the origin.
Also, 2640 chambers (3D-cells, facets), 7440 faces (2D-cells), 7200 edges (1D-cell).
(Each cell in a given category, 3D, 2D, 1D or 0D, has a center lying on a sphere of the same radius)
Euler's formula holds: 0=2640-2400+7440-7200
Among the 2D-faces, all are regular, there are 1440 pentagons, 3600 squares and 2400 triangles.
The edges are of two types: 3600 are on the side of a dodec, 3600 on the side of a tetra.
The following table gives information on the chambers:

Chamber type	Image	color in the pictures	Total number	Number touching one vertex
Regular dodecahedron		green	120	1
Prism with regular pentagonal base and square sides		purple	720	3
Prism with equilateral base and square sides		yellow	1200	3
Regular tetrahedron		orange	600	1

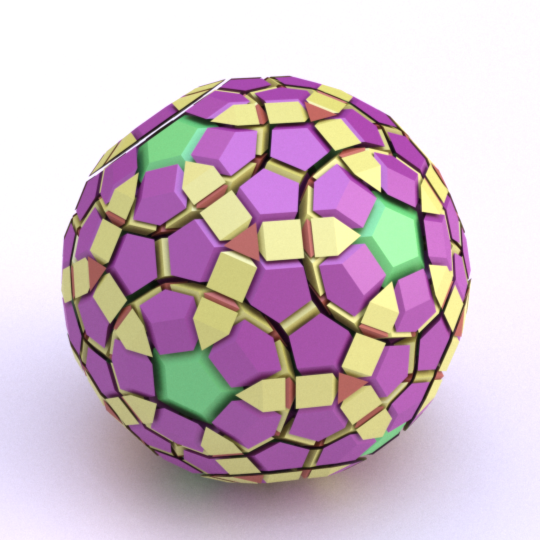For the representation, it was projected orthogonally to R³ and then rendered into a 2D image. We used LuxRender for a photorealistic look. For the projection from R⁴ to R³, we chose one putting a dodecahedron at the center, yielding an object with a lot of symmetries (as many as the regular dodecahedron). Some cells are projected flat (they are not represented on the picture on the left). All others come in pairs that have exactly the same projection. You may look at this webpage for many nice pictures of this object, and more info. Many other polytopes can be found on that webpage.